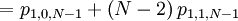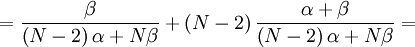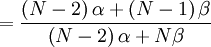Price 3.0
From Evolution and Games
(→A B mutant in a population of A's) |
(→A B mutant in a population of A's) |
||
| Line 115: | Line 115: | ||
This is pretty well defined, so we can also compute the covariance of these two actual random variables. Let us start with the expectations... | This is pretty well defined, so we can also compute the covariance of these two actual random variables. Let us start with the expectations... | ||
| + | <math>\mathbb{E}\left[ X\right] =\frac{N-1}{N}</math> | ||
| + | |||
| + | <toggledisplay> | ||
| + | <math>\mathbb{E}\left[ X\right] =\sum_{x=0,1}x\mathbb{P}\left( X=x\right) = </math> | ||
| + | |||
| + | <math>=\mathbb{P}\left( X=1\right) = \frac{N-1}{N}</math> | ||
| + | </toggledisplay> | ||
| + | |||
| + | |||
| + | For symmetry reasons... | ||
| + | |||
| + | <math>\mathbb{E}\left[ Y\right] =1 </math> | ||
| + | |||
| + | |||
| + | <math>\mathbb{E}\left[ XY\right] = \frac{\left( N-2\right) \alpha +\left( N-1\right) \beta }{\left(N-2\right) \alpha +N\beta }</math> | ||
| + | |||
| + | <toggledisplay> | ||
| + | |||
| + | <math>\mathbb{E}\left[ XY\right] =\sum_{x=0,1}\sum_{y=0}^{N}xy\mathbb{P}\left(X=x,Y=y\right) \text{ (because }xy=0\text{ if either }x=0\text{ or }y=0\text{)} </math> | ||
| + | |||
| + | <math>=\sum_{y=0}^{N}y\mathbb{P}\left( X=1,Y=y\right) = </math> | ||
| + | |||
| + | <math>=\sum_{k=0}^{N}y\mathbb{P}\left( X=1\right) \mathbb{P}\left(Y=k|X=1\right) = </math> | ||
| + | |||
| + | <math>=\frac{N-1}{N}\sum_{k=0}^{N}\left[ \frac{1}{N-1}k\binom{N}{k}\left(p_{1,0,N-1}\right) ^{k}\left( 1-p_{1,0,N-1}\right) ^{N-k}+\frac{N-2}{N-1}k\binom{N}{k}\left( p_{1,1,N-1}\right) ^{k}\left( 1-p_{1,1,N-1}\right) ^{N-k}\right] </math> | ||
| + | |||
| + | <math>=p_{1,0,N-1}+\left( N-2\right) p_{1,1,N-1} </math> | ||
| + | |||
| + | <math>=\frac{\beta }{\left( N-2\right) \alpha +N\beta }+\left( N-2\right) \frac{\alpha +\beta }{\left( N-2\right) \alpha +N\beta }= </math> | ||
| + | |||
| + | <math>=\frac{\left( N-2\right) \alpha +\left( N-1\right) \beta }{\left(N-2\right) \alpha +N\beta }</math> | ||
| + | </toggledisplay> | ||
| + | |||
| + | Thus.... | ||
| + | |||
| + | <math>Cov\left( X,Y\right) =\mathbb{E}\left[ XY\right] -\mathbb{E}\left[ X\right] \mathbb{E}\left[ Y\right] =\frac{\left( N-2\right) \alpha +\left( N-1\right)\beta }{\left( N-2\right) \alpha +N\beta }-\frac{N-1}{N} </math> | ||
Revision as of 22:11, 18 September 2010
A model with frequency dependence
We go back to the asexual model, but now we will take a model with frequency dependence. This will be a bit more complicated, but we can still use a basic, well known model. Suppose there are $N$ individuals. They are paired randomly, and play a game in those pairs. The game gives them payoffs according to the following payoff matrix
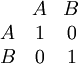
If qi = 1, then that means that individual $i$ plays strategy A, and qi = 0 means that individual i plays strategy B. Depending on the match, each individual gets a payoff πi. The next generation is drawn, as before, one individual at a time. But now the probability that i is drawn as a parent depends on the payoff, and not just on the own genotype.
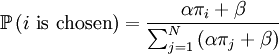
We do the same procedure as before, but with a few different starting points.
An A mutant in a population of B's
Suppose q1 = 1 and qi = 0 for i = 2,...,N. The probability of any individual i = 2,...,N to be matched with individual 1 is 1 / (N − 1).
The probability with which an individual i with qi = 1, who is matched to an individual j with qj = 0, is chosen is:
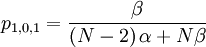
The probability with which an individual i with qi = 0, who is matched to an individual j with qj = 0, is chosen is:
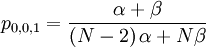
The probability with which an individual i with qi = 0, who is matched to an individual j with qj = 1, is chosen is:
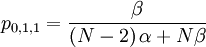
We can also compute the covariance of these two actual random variables. Let us start by computing expectations...
![\mathbb{E}\left[ X\right] =\frac{1}{N}](/wiki/images/math/c/3/8/c389f3bfffa4dec33da0820781e6e99b.png)
For symmetry reasons...
![\mathbb{E}\left[ Y\right] =1](/wiki/images/math/f/6/8/f6851309af9717e71df00c1227f0d760.png)
![\mathbb{E}\left[ XY\right] =\frac{\beta }{\left( N-2\right) \alpha +N\beta }](/wiki/images/math/b/c/1/bc1ba1eb3290d07e011aad2aaf8c6036.png)
The Covariance is thus...
![Cov\left( X,Y\right) =\mathbb{E}\left[ XY\right] -\mathbb{E}\left[ X\right] \mathbb{E}\left[ Y\right] =\frac{\beta }{\left( N-2\right) \alpha +N\beta }-\frac{1}{N}](/wiki/images/math/4/5/1/451efdc1824fac07a0b1bf56a8167a29.png)
A B mutant in a population of A's
Suppose q1 = 0 and qi = 1 for i = 2,...,N. The probability of any individual i = 2,...,N to be matched with individual 1 is 1 / (N − 1).
The probability with which an individual i with qi = 0, who is matched to an individual j with qj = 1...
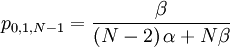
The probability with which an individual i with qi = 1, who is matched to an individual j with qj = 1...
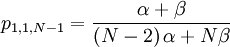
The probability with which an individual i with qi = 1, who is matched to an individual j with qj = 0...
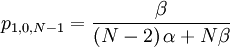
So...
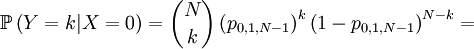
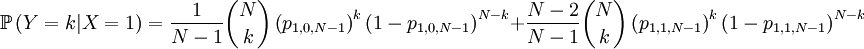
This is pretty well defined, so we can also compute the covariance of these two actual random variables. Let us start with the expectations...
![\mathbb{E}\left[ X\right] =\frac{N-1}{N}](/wiki/images/math/8/a/9/8a9d1397815d5042bde4b9407714f7da.png)
For symmetry reasons...
![\mathbb{E}\left[ Y\right] =1](/wiki/images/math/f/6/8/f6851309af9717e71df00c1227f0d760.png)
![\mathbb{E}\left[ XY\right] = \frac{\left( N-2\right) \alpha +\left( N-1\right) \beta }{\left(N-2\right) \alpha +N\beta }](/wiki/images/math/a/1/b/a1b3f65cc47eb865d7eaed9b55f193af.png)
Thus....
![Cov\left( X,Y\right) =\mathbb{E}\left[ XY\right] -\mathbb{E}\left[ X\right] \mathbb{E}\left[ Y\right] =\frac{\left( N-2\right) \alpha +\left( N-1\right)\beta }{\left( N-2\right) \alpha +N\beta }-\frac{N-1}{N}](/wiki/images/math/a/f/e/afe717cf465e229083a9af7442bd1559.png)
![\mathbb{E}\left[ X\right] =\sum_{x=0,1}x\mathbb{P}\left( X=x\right) =](/wiki/images/math/5/c/8/5c8589cd4e69154ab8f56f7db9f0dc8f.png)
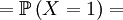
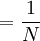
![\mathbb{E}\left[ XY\right] =\sum_{x=0,1}\sum_{y=0}^{N}xy\mathbb{P}\left(X=x,Y=y\right) \text{ (because }xy=0\text{ if either }x=0\text{ or }y=0\text{)}](/wiki/images/math/f/1/4/f146e34f77cefe890894be133473bf2a.png)
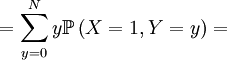
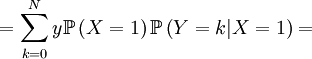
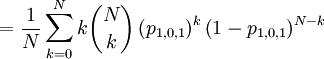
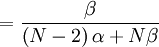
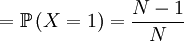
![=\frac{N-1}{N}\sum_{k=0}^{N}\left[ \frac{1}{N-1}k\binom{N}{k}\left(p_{1,0,N-1}\right) ^{k}\left( 1-p_{1,0,N-1}\right) ^{N-k}+\frac{N-2}{N-1}k\binom{N}{k}\left( p_{1,1,N-1}\right) ^{k}\left( 1-p_{1,1,N-1}\right) ^{N-k}\right]](/wiki/images/math/7/4/9/749b567e6d87135a52d34cb2787efab1.png)